Using Lattice Green Functions to Solve Poisson's Equation

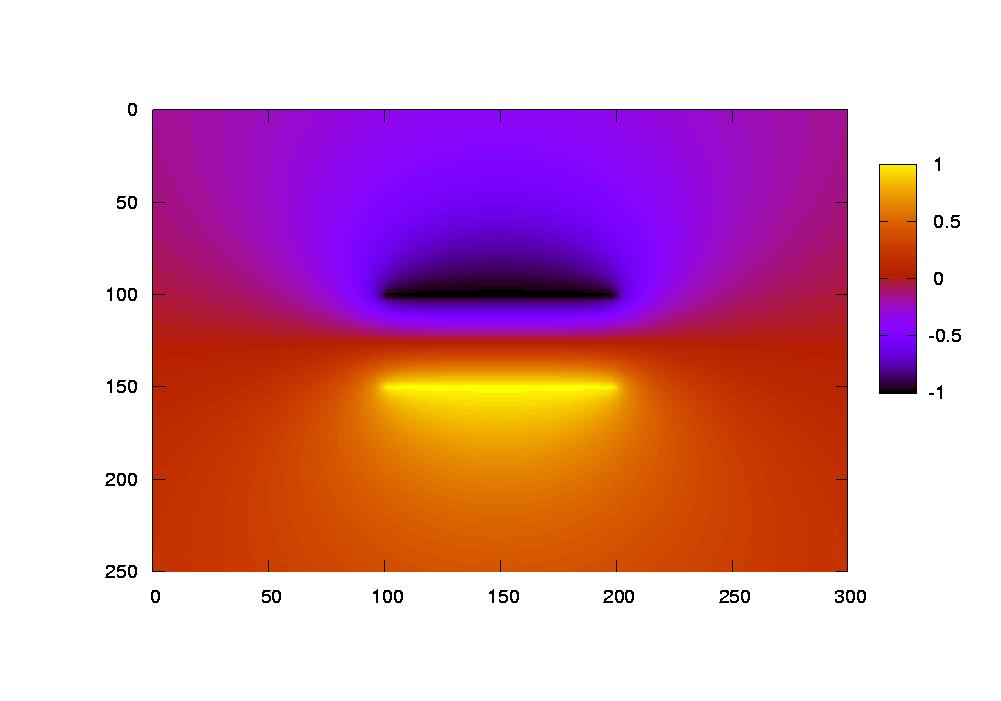
Here is a potential plot of a parallel plate stripline consisting of 100 charges per plate with the distance between the plates being half the width of the plates. The positive plate is on the bottom.

Here is a closeup of the left half of the bottom plate (positive plate) of the parallel plate stripline shown above.

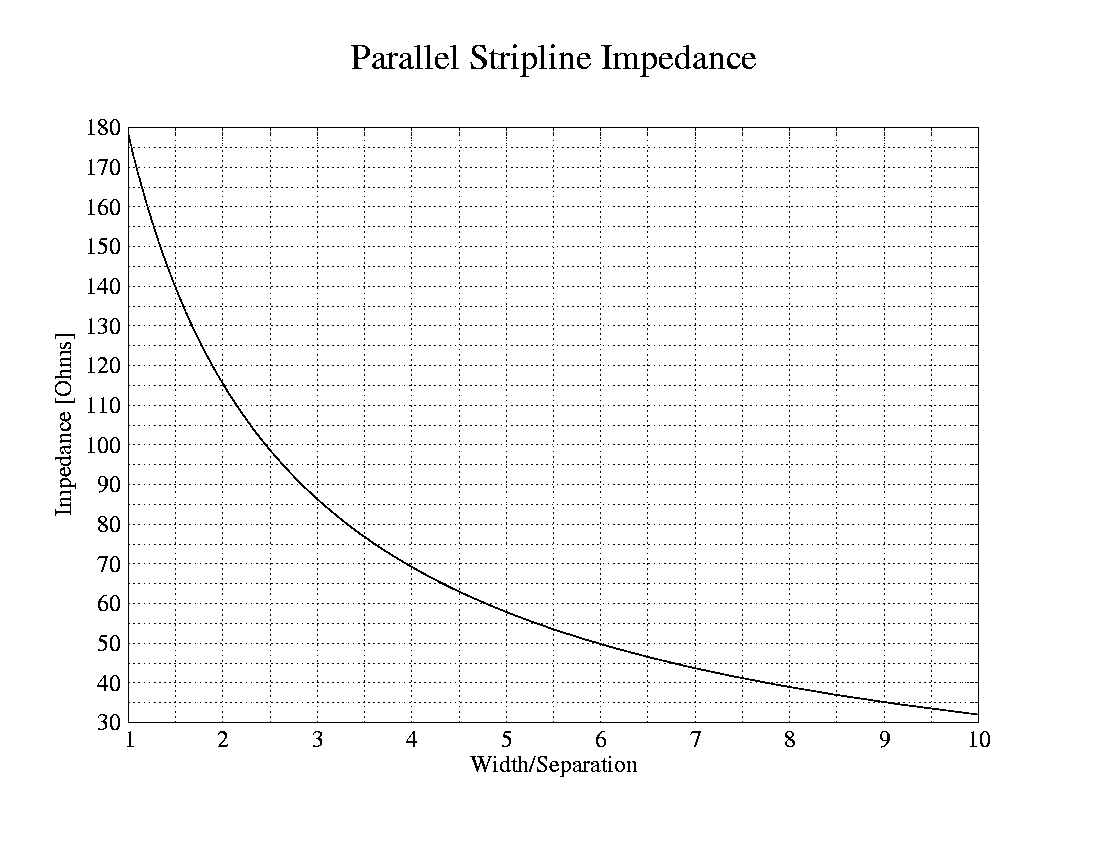
Here is a plot of impedance versus width/separation for parallel plate striplines calculated the LGF way using a stripline width of 1000 and a separation of 100...1000.

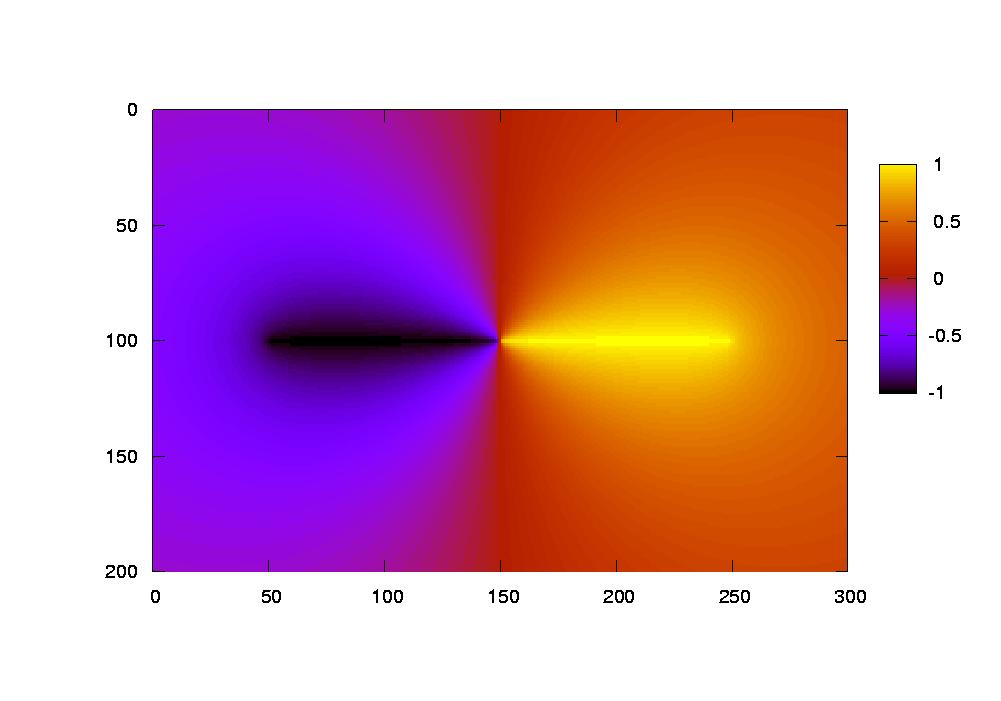
Below are images of potential plots of a coplanar stripline.

Here is a coplanar stripline with 100 charges on each plate and a separation of zero lattice points between plates.

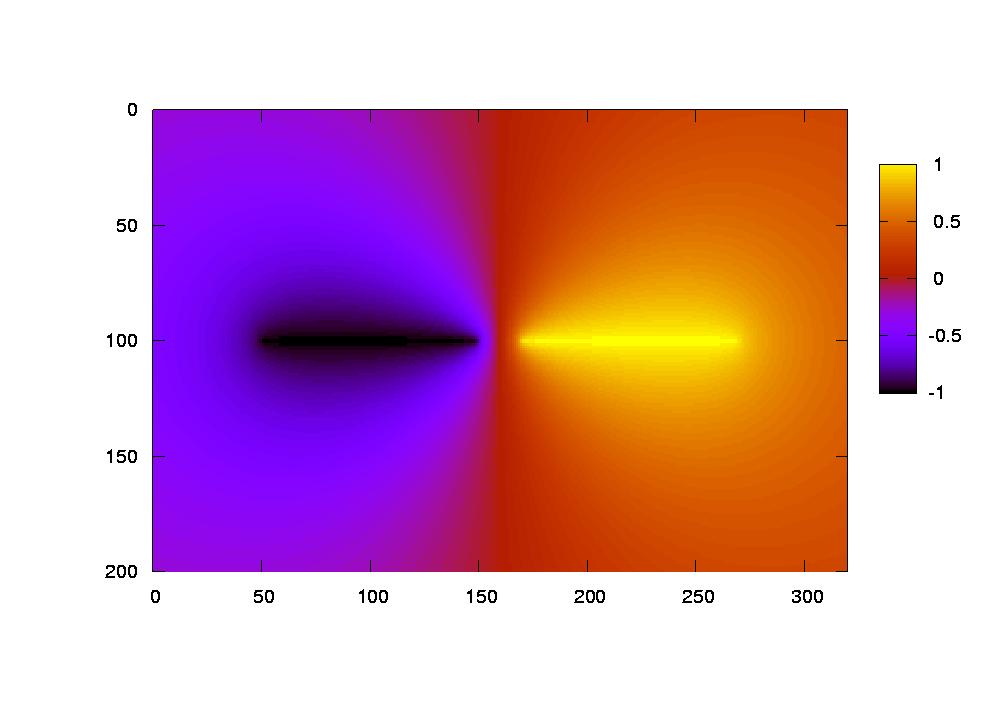
Here is another coplanar stripline also with 100 charges on each plate, but with a larger separation of 20 lattice points between plates.

Below is an image of the potential of a right angle stripline with 100 charges on each plate, with each plate starting one lattice point from the origin and lying along the positive x or y axis.

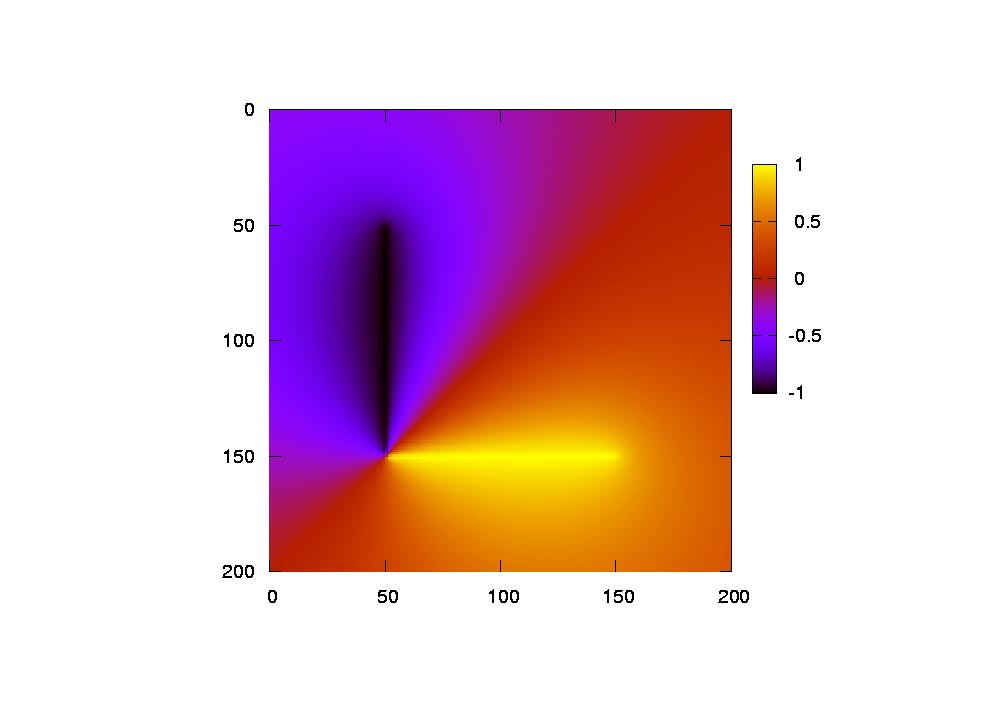
Here is a potential plot of a single +1 charge in the middle of a square box whose sides are at zero potential with 100 charges on each side. Note that this is a charge neutral system, with all charges on the sides of the box adding to -1, so that the potential outside of the box is zero everywhere. Simple contour lines are used here instead of color shading to show more clearly how the circular potential lines near the center charge morph into a square as we get nearer to the box sides.

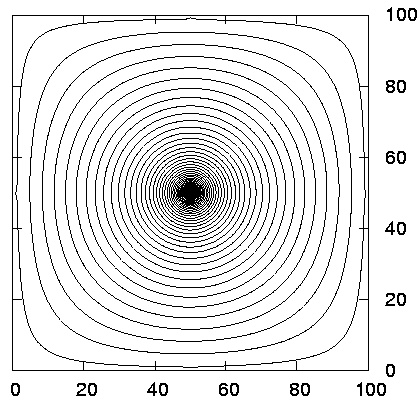
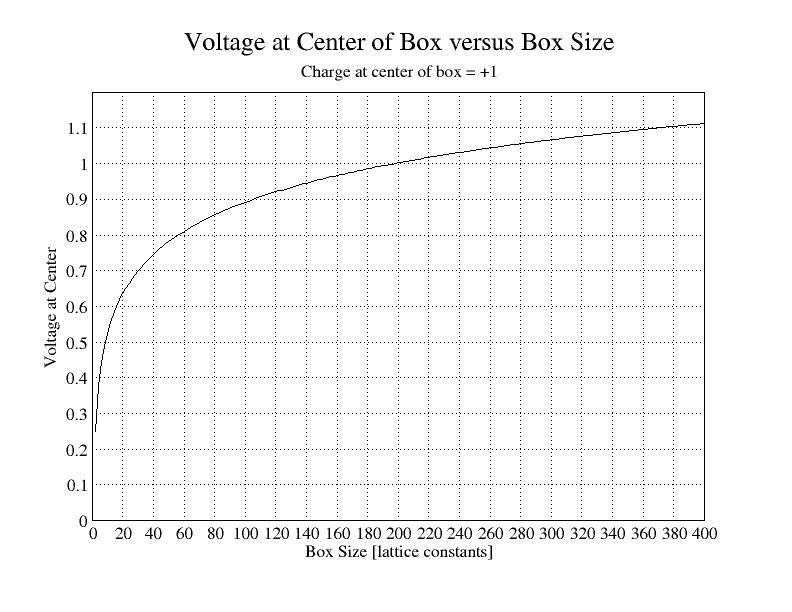
Below is a plot of the voltage at the center of the square box, versus box size in units of lattice constants, for the system as described above. The voltage is logarithmically divergent as the size of the box goes to infinity. A good approximation formula for the voltage is: V = 0.155695 + 0.159801 * ln( n ), where n is the box size.

Last modified Aug 12, 2005.

For more information contact:
Stefan Hollos
stefan[AT]exstrom DOT com
(303) 678-1487